An MS Word version of this document (for printing/viewing)

1. (8 pts) Determine whether the relation below represents a function. What is the domain and what is the range?

{(-3,6), (7, 5), (4, 9), (7, 5)}

2. (5 pts) Determine whether the equation defines y as a function of x. If it does not, show/explain why not.

3. Let . Determine the following, if possible. If not possible, state why:

a. (2 pts)

b. (2 pts)

c. (2 pts)

4. (7 pts) Find the domain of .

5. (4 pts) Let . Find the average rate of change of f from x = -1 to x = 1.

6. Let and .

a. (5 pts) Determine the domain of f.

b. (5 pts) Determine the domain of g.

c. Find the following functions and state the domain of each.

i. (3 pts)

ii. (3 pts)

iii. (3 pts)

7. Determine algebraically whether each function is even, odd, or neither.

a. (2 pts)

b. (2 pts)

8. Use the graph of the function f, below, to find:

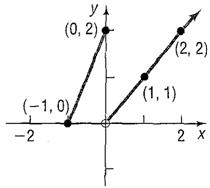
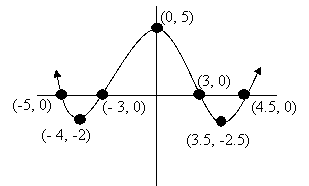a. (5 pts) The intercepts (Express answers in ordered pairs.

b. (5 pts) The domain and range.

c. The local extreme points (Give actual points on the graph.)

i. (2 pts) f has a local maximum at

ii. (2 pts) f has local minima at

d. The intervals on which f is increasing, decreasing, or constant.

i. (2 pts) f is increasing on

ii. (2 pts) f is decreasing on

iii. (2 pts) f is constant on

9. Graph each of the following functions using the techniques of shifting, compressing, stretching, and/or reflecting. Start with the graph of the basic function and show all stages.

a. (6 pts)

b. (7 pts)

10. (8 pts) Sketch the graph of . Include all intercepts. State the domain and range.

11. (6 pts) Determine the piecewise-defined function g from its graph, below.